FACTS ABOUT THE SAVINGS OF FOSSIL FUEL
BY WINDTURBINES IN THE NETHERLANDS1.

C. (Kees) le Pair


Abstract.

Electricity production in The Netherlands using renewables, especially wind,  has grown to a size that makes it visible in the national statistics of electricity generation. Its influence on fossil fuel consumption can be determined. Based on these 'official figures' we show the actual contribution of fuel reduction to be equivalent to about 4,1% of the installed - 'nameplate' - capacity.
The actual data also provide some insight into the mechanism that causes wind electricity to have such a dramatically small influence on primary fuel consumption.



Introduction
Renewables are being introduced into  electricity generation in order to save fossil fuel and to reduce the amount of CO2 emissions. In an early stage of this process a simple supposition convinced authorities and the public at large of the effectiveness of using renewables. A kWh of electricity produced free of CO2, would replace a kWh conventionally produced. It would therefore save the amount of the fossil fuel otherwise needed to produce that same quantity of electricity.
This simple notion has been criticized. The renewable electricity generation influences the way conventional units operate which in turn reduces their efficiency, and actually results in less savings. Conventional units are necessary when some of the renewable resources like wind and PV solar, are not available. They come when the wind blows or only during daylight hours. Electricity storage, which would alleviate this problem, is only feasible in very special situations. Otherwise it is too expensive.
World wide, a debate has evolved between the protagonists of large-scale renewable systems and critics who argue that the systems do not function as promised. Because quite substantial amounts of money are involved, there is a lot at stake. Many people, whose incomes and occupations are linked to the renewable sector do not appreciate the arguments put forward by the critics. Politicians, who have tried hard in the past to push renewables, risk damage to their green image if they suddenly change sides.
The dispute has continued for some time because of inherent difficulties in determining the decisive facts that would facilitate objective decision making. First of all, the electricity systems in different countries and regions are not alike. Therefore the way in which intermittent sources influence the overall operation differs from country to country. Thus, arguments that are valid in one place may not, or not as equally well, influence matters elsewhere. Secondly there is reluctance among producers to reveal the relevant production data. They claim that it is competitively sensitive data. Also we must realize that some of the data necessary to make final conclusions are simply not available. Thirdly, as some of the renewables advocates say, present day figures are not conclusive because the overall system is changing; for instance stronger interconnections between regions and so-called smart grids may improve the situation.

We took an active role in this debate as can be read in a number of earlier contributions (2,3...9). We have been in contact with other investigators abroad, whose work helped us to better understand the complexity of the problem and strengthened our conviction that there is something fundamentally wrong with today's large scale renewable development. To mention but a few, I'd ask the reader to review the work on developments in the USA by W. Post (10) and on Denmark by H. Sharman (11) and P.F. Bach(12). There are many more excellent contributions and I apologize to their authors for not listing them all. Due to the lack of all the necessary data many studies have been based on models filled in with available, though incomplete, data and topped up with general knowledge about systems and components.
Noteworthy exceptions are the studies by BENTEK on the electricity systems of Texas and Colorado (13), of F. Udo on the Irish case (5, 7, 9) and several studies about Denmark (11, 12).
BENTEK used the actual pollutants' emission data to show that adding wind did not achieve the objective of reducing the emissions. Udo used the detailed production time series provided by the Irish grid operator EIRGRID to show that wind accomplished much less than previously assumed. In Denmark the wind penetration is so strong that the results show up in all kinds of national statistics. They reveal that the Danes can use only half of their wind made electricity. They face other disadvantages too. For instance, they pay about twice as much for electricity as the French do.
However, these facts on foreign systems are not sufficient to influence policy at home. The argument that situations and systems are not alike and may not be easily compared provide an all too convenient veil to hide behind.
We have summed up most of the critical arguments in a recent article in EurophysicsNews (8). In a previous paper, I presented a model of a hypothetical wind turbine assembly in the center of the Netherlands using actual wind behavior and known properties of gas-fueled backup generation to show that wind electricity might even increase fuel use (6). But, of course, a model is not as convincing as actual facts.



Electricity production in the Netherlands
The Netherlands Central Bureau of Statistics collects data on electricity production. It composes annual time-series which are publicly available on its website 'Statline' (14). At present the relevant series cover the years 1998 - 2010. Some of the data of 2010 are still labeled 'provisional'. But the actual figures that are expected later in the summer of 2012 will not differ too much from those already published. They will therefore not influence the trends we have computed and used below.
Renewable production has now reached a high enough level to show up in these macro statistics. We have analyzed them and present the results here. We produced graphs from which we derived the current trends. In our calculations we used these trend results. Because there is some spread in the data, actual values for a certain year may differ somewhat from the ones used. The graphs we present will show how much uncertainty this implies.
Throughout this contribution we use GWy (Gigawattyear) as the unit of electric energy and caloric energy. Power is expressed in GWe (Gigawattelectric) (15) .

Table 1

Contribution to the national electricity production in 2010 from trends.

primary
energy
source		 production
[GWy]		 [%]
of
total		 annual
increase
[GWy]		 specific
growth p.a.
[%]
national production
fossil production
nuclear production
renewable energy

natural gas
coal
oil
other fossil

biomass
wind
hydro power
solar (PV)

other energy
carriers
13,03
11,00
0,46
1,26

7,80
2,62
0,01
0,49

0,73
0,49
0,011
0,006

0,34
100,00
84,42
3,53
9,67

59,86
20,11
0,08
3,76

5,60
3,76
0,08
0,05

2,52
0,252
0,155
0,002
0,091

0,177
-0,013
-0,004
-0,0049

0,0492
0,0412
-0,0001
0,0005

0,0042
1,89
1,41
0,43
7,22

2,27
-0,50
-40,00
-1,00
6,74
8,37
-0,89
8,06

1,25

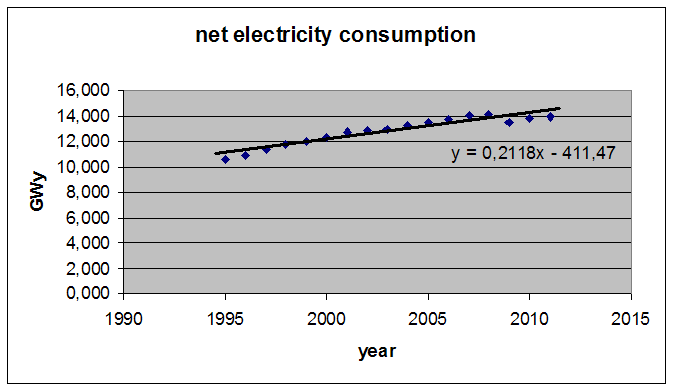
During the whole period domestic demand exceeded national production. The Netherlands was a net importer of electricity. The situation is changing however. Capacity was augmented and we expect  the country to be a net exporter of electricity by 2012. We depict some characteristics in the next four graphs.

1. national production capacity 2. national electricity consumption
3. fossil production 4. renewable production

Notes:

  • Average capacity growth over the past four years ≈ 0,9 GWe p.a.

  • Consumption growth ≈ 0,212 GWy p.a.

  • A substantial part of the electricity production is accompanied by heat production. This heat is used for industrial purposes, process heat, green houses and domestic heating. It enhances useful utilization of primary energy, although it slightly reduces the efficiency of electricity production.

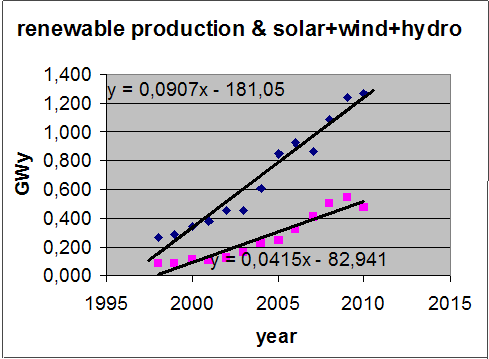
  • Renewable production includes wind, solar and hydro; these three together are also depicted separately. Actually the renewable electricity in the Netherlands is derived almost entirely from biomass and wind.

  • The growth of fossil+renewable production (0,246 GWy p.a.) exceeds the consumption growth. Net imports diminish.

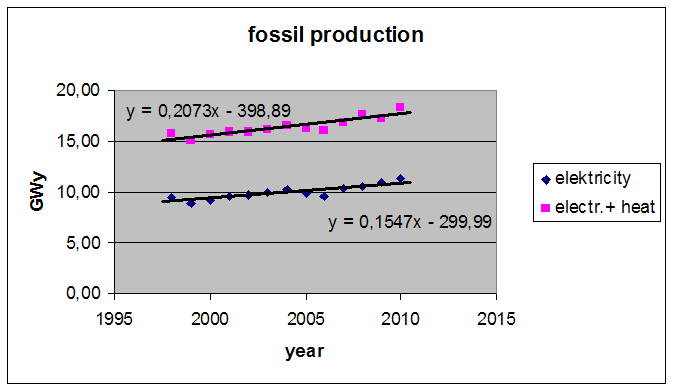
  • The growth of fossil production is entirely due to increase of natural gas, see table 1. However, in the year 2012 there is quite some new coal capacity under construction.

  • Another characteristic is decentralized production. About 8 GWy is produced by traditional electricity companies. Another 5 GWy is made in a decentralized manner by industrial and other companies and by agricultural firms.


Efficiency
Efficiency is a crucial issue with electricity production. The CBS offers data about fuel consumption in caloric units (TJ) for the different fuels used. There are also data about the fuel consumption of different installations. But analysis is complicated by the fact that nowadays many generating units use fuel mixes. E.g. Steam turbines, once only coal fired, now have extensions for gas. Some Open Cycle Gas Turbines (OCGT) use waste industrial gas, which they top up with natural gas. And even the dominant generating type of Combined Cycle Gas Turbines (CCGT) may get some primary energy from burning coal. Efficiency of conventional electricity generation, η, is defined as:

η = (amount of electricity produced)/ (input of caloric heat)

Windturbines convert wind energy directly into electric energy. There is a theoretical value for the fraction of wind energy a turbine can extract under favourable conditions. However, when the wind is not strong enough, the turbines produce less or even nothing at all. When the wind is too strong the turbines have to be stopped, also then there is no production. Therefor a capacity factor is used. It is defined as:

CF = (electricity produced during a whole year) / (theoretical production with optimal wind)

We present some of the relevant CBS data in the next series of graphs.

5. efficiency total fossil fuels 6. capacity factor all wind turbines
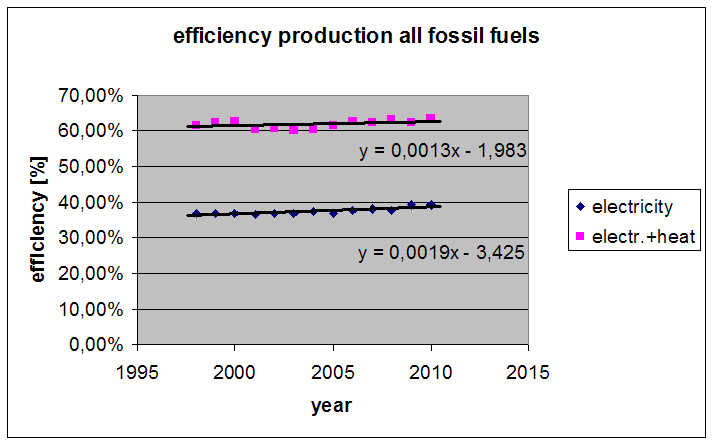
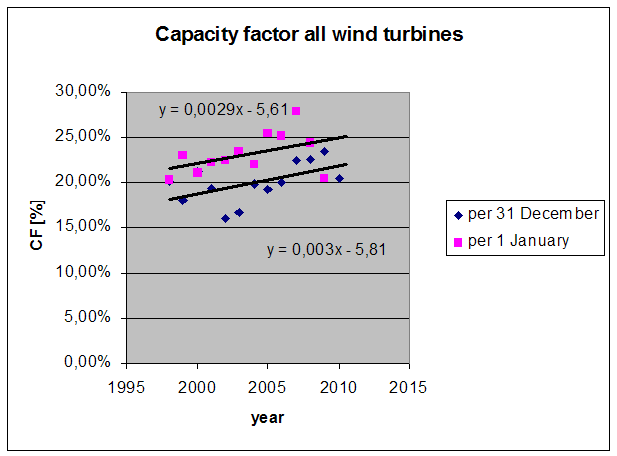
7. coal efficiency 8. natural gas efficiency

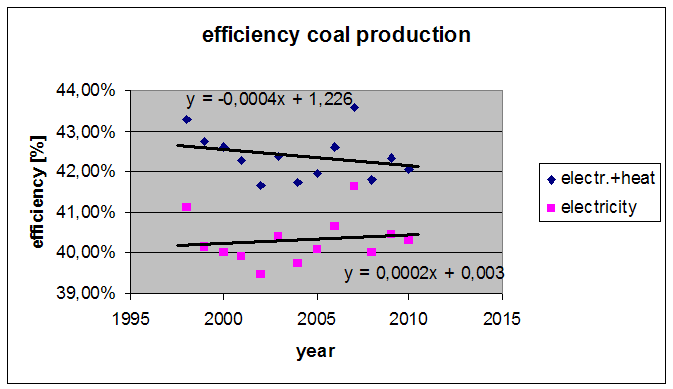
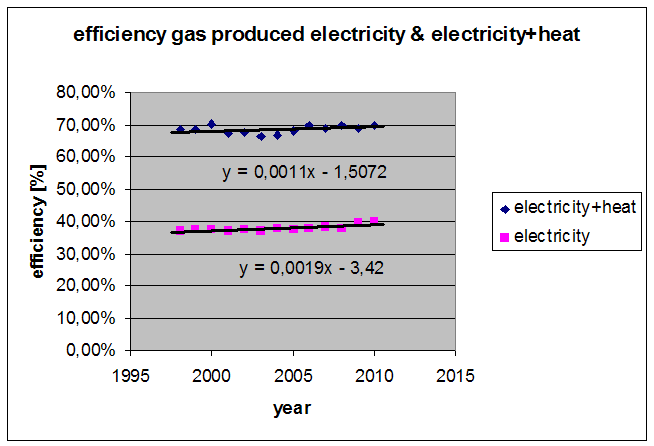
Notes:

  • Wind generating capacity grew fast. The exact time of the start of operations of new developments is unknown. Therefore we calculated CF using the installed capacity at the beginning and at the end of a year. The real value is somewhere in between. Currently about 23% for all together.

  • The trend  of η(coal) change is small compared to the scatter of the data. We consider this insignificant.

  • There is more to say about the efficiency of gas. First of all we notice a remarkably low electric efficiency - even lower than that of coal production. Although there is a slight and steady improvement, this is nevertheless lower than we expect; see below.


Efficiency anomaly
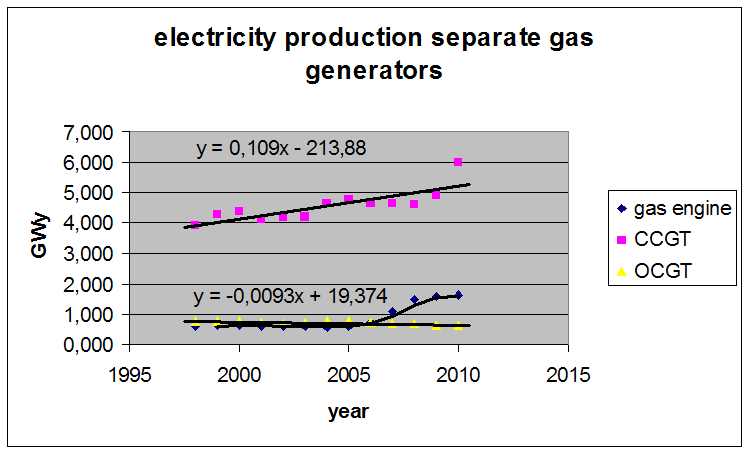
The electricity production using natural gas is mostly by CCGTs. Then there is a contribution by gas engines and finally by OCGTs. The separate contributions are depicted in figure 9.

    9. production by different types of gas-fueled generators
Properties of the dominant generators in the Netherlands when operating under design conditions are as follows:

  • CCGT. For older types η ≈ 0,55. The newest types have η ≈ 0,60. The capacity has been extended by adding the more efficient types. Over the whole period one might expect about 40% replacement by the newer types. The present average efficiency is therefore estimated to reach η ≈ 0,59 (16).

  • The steam turbines are mainly coal fired. Their η ≈ 0,455 (16).

  • The OCGT number shows some increase, although the intention is to use them less because of their low efficiency, which have η ≈ 0,32 (16).

    • One can speculate about the reasons for adding yet more OCGT generators. One reason could be the need to compensate for rapid variations caused by windpower. They are also used in connection with low caloric waste gas of the steel mills. Given that the steel mills have not increased in capacity,  this is therefore not the driver to  install more of them.

  • The gas engine is popular among small industrial companies and the agricultural sector. η varies much with the size: ≈ 0,26 – 0,45   (17).

  • The nuclear reactor shows a stable behavior. After a renovation around 2007 a steady η of about 0,348 rose stepwise to 0,377(18) where it remains.

No generator works all year round at design capacity. Repairs and normal maintenance prevent this. Taking this into account the data show that coal fired generation and the nuclear installation perform as expected. They are used as 'must run' units providing power in the range not affected by daily or even seasonal variations. The difference between their actual performance and the theoretical one under design conditions is small. It can be attributed to these normal maintenance windows stops and some minor contribution to demand variations.
For the gas segment this is different. Taking into account the numbers of different generator types, we would expect η to be 0,51. The actual efficiency according to the national CBS data is ≈ 0,394, i.e. about 12% less. This cannot be explained by repairs and maintenance alone. Here we see the influence of ramping: lowering and raising the production and of multiple stops and start-ups in order to cope with variations in demand and variations in supply by the increase in irregular production such as wind power.
In former discussions with the responsible Minister we were told that these variations would not adversely affect the efficiency by more than 1 or 2%. The actual data show that these effects have much more influence. Stops and starts and steep ramp ups/downs influence the efficiency much more than 2%. This raises the question what the effect of wind electricity really is and how much it impacts the consumption of fossil fuel?


Fossil fuel saving by renewables
In order to compute the efficiency with which electricity produced by renewables saves fossil fuel, we write the national production, E(t), as sum of its components:

E(t) = ∑ Ei(t)

where: i = f (fossil-fuel produced electricity), n (nuclear ibid), r (by renewables ibid), o (by other energy carriers ibid).
From the CBS data we derive the trends over the period 1998 - 2010:

    Ef(t) = 0,1547 x Yr – 299,99 (fig. 3)
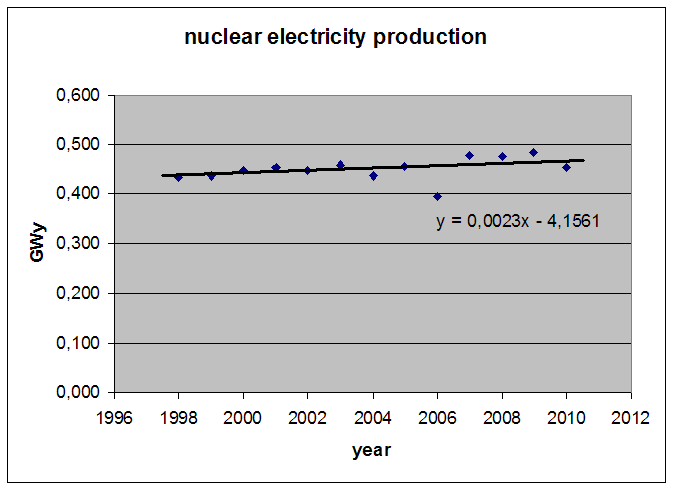
    En(t) = 0,0023 x Yr – 4,1561 (fig. 10 below)
    Er(t) = 0,0907 x Yr – 180,5 (fig. 4)
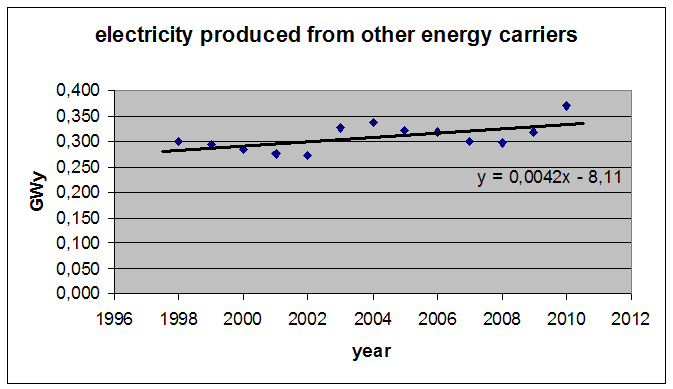
    Eo(t) = 0,0042 x Yr – 8,11 (fig. 11 below)
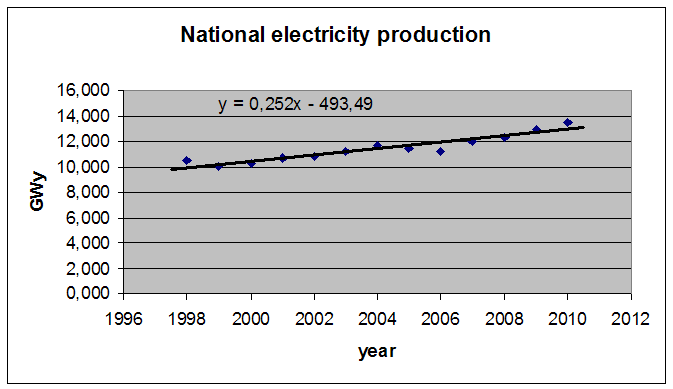
    E (t) = 0,252 x Yr – 493,49 (fig. 12 below)

Yr = 1998, 1999, ..., 2010.
The unit of energy = GWy.
NB. Adding the four components would result in:

    E (t) = 0,2519 x Yr – 492,7561

The difference is due to rounding though is not visible in the graph.
The electricity production is growing (dE(t)/dt). The annual increment being ΔE = 0,252 GWy.

ΔE = ∑ ΔEi

    ΔEf = 0,155 GWy
    ΔEn = 0,002 GWy
    ΔEr = 0,091 GWy
    ΔEo = 0,004 GWy

In order to realize the same growth without renewable contribution, the fossil contribution would have to increase: ΔE'f = 0,155 + 0,091 = 0,246 GWy. The incremental saving of fossil fuel by renewables is due to 0,091 GWy of electric energy. If this would be 100% effective, we would see a caloric saving equal to 0,091 / ηe,f(t).
The CBS data let us calculate ηe,f:

    ηe,f(t) = 0,0019 x Yr - 3,425 (fig. 5)

yielding: ηe,f(2010) = 0,394. As a result this fuel saving would be equivalent to its caloric content: 0,091 / 0,394 = 0,231 GWy.
The CBS data also allow us to calculate what this contribution actually does. The consumption of fossil fuel, If(t), is among the Statline data provided:

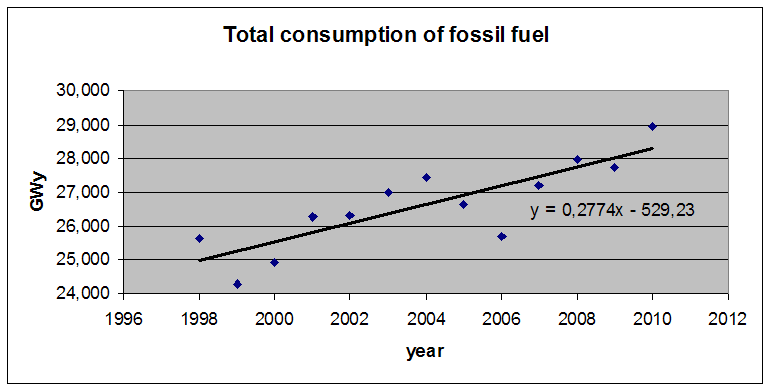
    If(t) = 0,2774 x Yr - 529,23 GWy (fig. 13 below)

This means that at present the system needs an annual increment of fuel equivalent to:
ΔIf(2010) = 0,2774 GWy. Under the same circumstances an increase of fossil produced electricity of 0,246 GWy in stead of 0,155 GWy would require:

    ΔIf'(2010) = 0,246 x 0,277 / 0,155 = 0,440 GWy

which is 0,440 - 0,277 = 0,163 GWy more than at present.
The 0,091 GWy renewable incrementally produced electricity therefore does not save 0,231 GWy but 0,163 GWy, which means it is 0,163 / 0,231 = 70,6% effective.

 

Please note: the validity of the differential approach to the whole of the developments rests on the data. All but the total capacity and the change in import/export (not shown) can be represented by linear trends. These two exceptions were not used in the calculations. Renewables were virtually not on the scene before 1998.
The 70,6% fuel saving efficiency is a property of the system. It does not depend on a single wind contribution increment. If in 2010 there had been no real wind increment and all of the renewable increment had been due to say for instance biomass, the result would have been the same (!). Even, though biomass does not, according to our assumption, substantially impair the fossil production. The generator assembly is a complex system. Many things influence its performance. For instance: the demand and its variations, the skill of the operators, the addition of new generators and the disappearance of older types and so on, and so on. One of them is the contribution of the existing wind turbines with their variations, growth etc. We described the total system with a number of trends of some overall parameters. Such a description is a symplification, although the outcomes are valid as long as the trends are stable. The trends over the twelve year period were remarkably stable and linear. A linear stable trend of fossil fuel use combined with a similar trend of fossil electricity production does not mean that all those units behaved the same way during these twelve years. Some units were replaced, some were used more, some less etc. It only means that from a macroscopic (national) view point there was a steady trend of these two parameters and a steady slow linear change in η. The latter probably due to a combination of the addition and renovation by new better performing units, also some less performing (gas motors) and of disturbing influences like the feed in of more variable wind electricity. There also was in the 1998 - 2010 period a steady linear change in wind capacity and wind electricity production. Other contributors of electricity to the grid are either negligible or remain virtually stable. Thus, they do not influence appreciably the behaveour of our fossil fueled generation.
As long as these trends persist one will arive at the same answers as derived above. If, however, starting for instance in 2010 the trend of an important parameter would change, then a change is to be expected in all important trends. But we would have to wait about 10 years to find the new trends and to do the calculations anew. Imagine that from 2010 on no new wind turbines would be added, we would have a system with a gradually deminishing wind contribution. This would have an effect on the performance of the gas fired system that does most of the backup and on η of the whole fossil assembly.
We would also see a difference in the quotient ηwhole system / ηincremental, which yields the present 70,6%.
In other words, the outcome of this paper would not change if for one year there would be no increment in wind electricity or if there would be quite an addition to the wind developments as long as it would not influence the trend lines. The increments are small compared to the whole of the existing system. But if a real break in trend lines would come about, then we would have different answers.

10. nuclear electricity production 11. electricity from other sources
12. national electricity production 13. consumption of fossil fuel



Fossil fuel saving by wind power
The electricity production of other renewables than wind and biomass is negligible. PV solar - another intermittent source, which could influence normal conventional production - contributes < 0,5% of all renewable production. Hydro makes about 1% and is not considered a disturbing contributor. So for the time being we deal only with wind and biomass, presently contributing 0,49 GWy and 0,73 GWy respectively.

Biomass. We have not investigated the merits and problems of biomass-produced electricity as thoroughly as we did with wind for obvious reasons. We suppose the conversion of liquid or gas fuel obtained from bio-material into electricity to be similar to the conversion of fossil fuels. If any problem exists, it is with the process of turning biomass into these fuels, i.e. a problem preceding the electricity production proper. There is no problem with random electricity supply variations. Bio-fuel can be stored. The generating equipment is also the same.
If biomass does have issues these are problems arising before it has been made into fuel. They are not visible in the Statline statistics dealing with electricity. One could think of costs - also energy costs - connected with collecting bio-waste, producing energy-rich crops, fertilizers, harvesting etc. There are other considerations regarding the competition with food production, acquiring soil to grow them and so on. We have studied the contents of a recent lecture on bio fuels by H.O. Voorma in which he outlines possibilities and difficulties of producing enough biomass for energy purposes (19). We understand there are quite some technological difficulties to tackle before biomass can be counted on in sufficient quantity to substantially fill our energy needs. We would add that it is necessary, as with other renewables, to do thorough energy studies of the whole chain in advance, before we decide to apply biomass on a large scale. If not, we cannot exclude that what is advocated as a solution, will turn out to be an expensive hoax, costing more in terms of energy than it delivers.
For the present study this is of no relevance. Bio-fuel is available in small quantities and it can be used in a conventional way to produce electricity. Apart from economic matters like costs, it is a matter of straightforward reliable operation based on known techniques. Therefore we assume that producing bio-based electricity is not different from that with fossil fuel. I.e. producing according to demand. This is a very important consideration, because it implies that adding bio electricity to the grid, does not impair the functioning and efficiency of other units.
Thus the reduction of the efficiency when adding renewable electricity to the grid, which we calculated in the preceding section, cannot be attributed to bio electricity. It must be solely due to the intermittent character of wind power (6).

The (trend) share of wind electricity equals 0,492 GWy, that of biomass: 0,732 GWy in 2010. Together this amounts to 1,224 GWy. 70,6% of this amount is actually saving fossil fuel. This reduces the saving of fossil produced electricity to 0,706 x 1,224 = 0,864 GWy. The addition of bio-electricity is assumed to be 100% effective. This leaves for wind: 0,864 - 0,732 = 0,132 GWy of electricity saving. This should be compared with the actual 0,492 GWy fed into the grid by wind developments. This leads to the conclusion:

Wind electricity is only 0,132 / 0,492 = 27% effective in fossil fuel saving.

Wind electricity. However, there is another catch, which does not show up in the Statline statistics. The construction of windturbines, their installation, grid adaptation and connection require considerable investments of energy. Some think these costs should not be taken into account because that is also not done for conventional plants. This is erroneous. Conventional plants are being installed to produce electricity according to societal demand. Wind turbines are not. They are being added to the system in order to save fuel and to diminish CO2 emissions. The question of whether they actually do therefore becomes essential. If not, they would only be superfluous supplements adding to the investment and other costs of the system.
The matter of energy costs associated with wind developments is another topic about which there is much dispute. The marketing blurb on wind electricity would have us believe that a wind turbine earns its energy investment back in about one half year of production. (Gross production, i.e. production taking into account the capacity factor.) This is contrary to our findings. According to research done by one of the main contractors installing windturbines in the Netherlands and abroad, it takes about 1,5 year to earn the energy investment in construction and installation back(20). Grid connection and adaptation plus extension of the high power grid was not included in this result. A more recent Australian study, analysed by F. Udo shows an earn back period of 2,8 year for Australia(21)(!). (In this study the energy costs of onshore grid expansion and adaptation were included. Offshore requires more energy. On the other hand distances in Australia are larger than in the Netherlands.)
For the energy involved in connection to the grid alterations in Western Europe we have no data. Let us therefore consider the additional capital investment in the grid in the Netherlands. Connecting wind developments to the grid in terms of money is almost as costly as the construction and installation of the turbines themselves. This is the case for onshore and offshore systems taken together. (Onshore, of course, is much cheaper, but offshore is a big issue.) In addition, the grid itself has to be re-enforced. In Germany recent estimates predict an extra 4000 km high-Voltage lines have to be installed to handle wind production. The problem of overproduction when there is adequate wind and the need to import electricity when there is a shortage, require regions to be connected. The Netherlands has for that purpose recently laid two under-sea cables, one to Norway and one to the UK. (The cable to Norway had already partly to be renewed after being in use for two years.) Combining all those adjustments we conclude that the 1,5 year 'earn back time' of the turbines alone should at least be doubled. Thus we count for 3 year 'earn back time'.
Another dispute continues about the expected lifetime of wind turbines and their additions. Promotors of wind turbines state a life time of 25 year. But the experience in the Netherlands is that wind developments had already to be renewed after only 12 year of operation. Sharman reported that the useful lifetime of wind turbines in Denmark is between 10 and 15 year (11). We would like to stick to facts, not to sales talk. So we prefer to discount these 3 year of earn back time over a useful lifetime of 15 year. This means that annually 20% of gross production must be subtracted from the net savings.



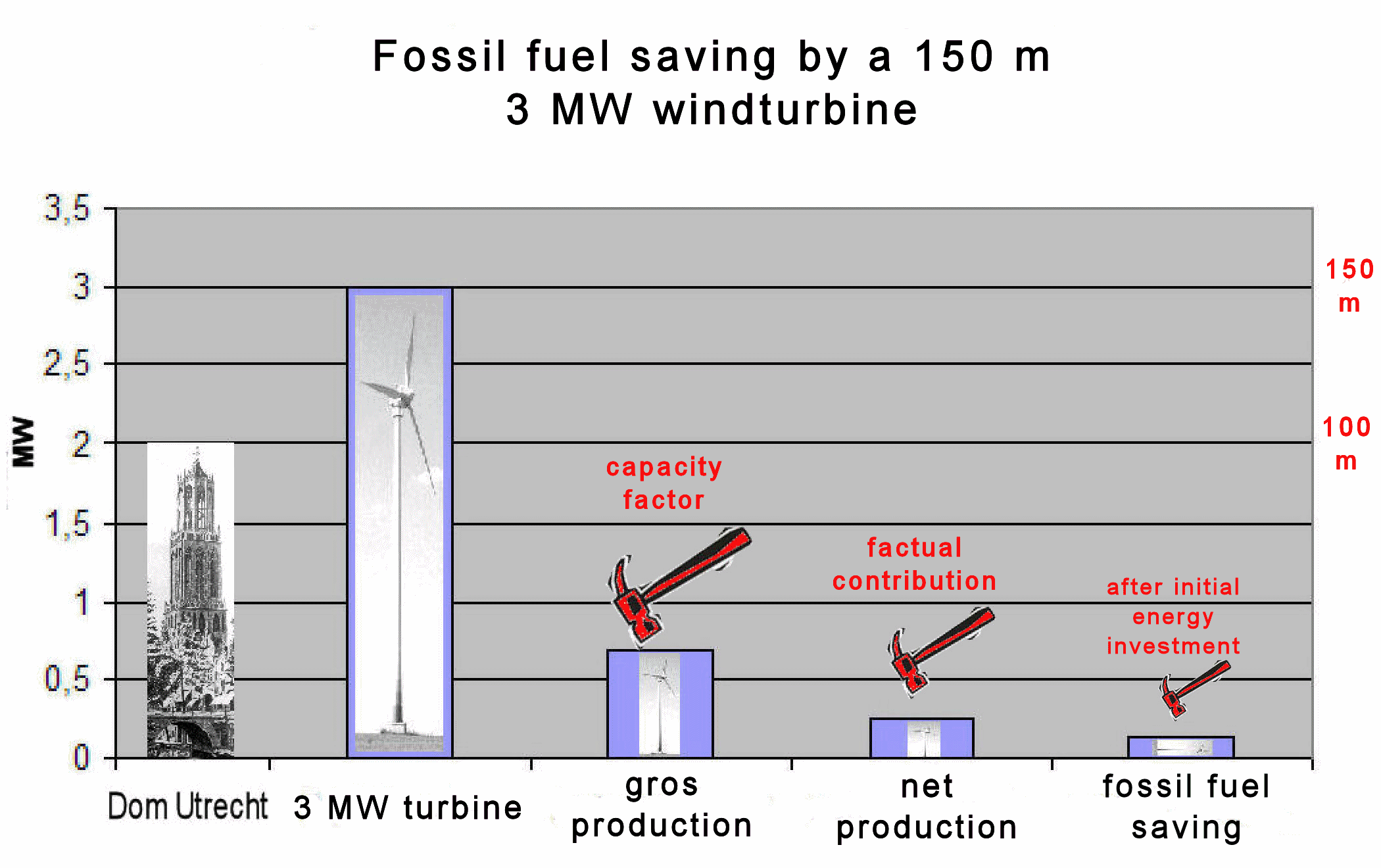
Conclusion and outlook
Adding it all up, one must conclude that under the present conditions in the Netherlands a 100 MW (Megawatt) 'name plate' capacity wind development produces on average 23 MW because of the capacity factor. 4,6 MW (20%) of this has to be subtracted from the final net result because of initial energy investments. From the actual Statline production figures we know that 27% of this 23 MW = 6,17 MW represents the actual fossil fuel and CO2 savings. But from this figure we need to subtract the amount of energy invested in the construction works: 4,6 MW. The net total of fuel saving electricity provided by our windturbines therefore is 6,17 - 4,6 = 1,57 MW on average over the year. That is ~ 1,6% of the installed capacity. It makes wind developments a Mega money pit with virtually no merit in terms of the intended goal of CO2 emission reduction or fossil fuel saving.

What is going to happen next? The current plan is to extend wind capacity to 8 GW onshore and 4 GW offshore. Presently wind name plate capacity is about 15% of the average domestic electric power need, which is roughly 14 GW. If the capacity exceeds 20% we enter into a new phase in which frequent curtailment sets in: there wil be periods in which the grid simply cannot absorb the supply. This situation already exists in Denmark and Ireland. Then we shall see a further dramatic decrease of the fuel-replacing effectiveness. In a previous study (6), we used a model in which the most conservative scenario had a thus defined windpenetration of 20%. We found that in that case savings were already negative, which means that wind developments actually caused an increase in fossil fuel consumption. The present study based on actual data shows that we are well on the way to reach that stage.

Nieuwegein,
August 28, 2012.

Change record
▪ Improvements in the description of how we arrived at
   the 'conclusion and outlook' inserted: August 22, 2012.
▪ G.Taylor drew attention to an arithmatic error. Correction
   reduced the difference between fossil produced electricity
   with and without wind to 0,163 GWy (previous version: 0,173).
   As a result wind effectivity falls to 1,6% (previous 4%).
   (Inserted: August 28, 2012).
▪ Following the advice of W. Post, K. Hawkins, G. Taylor &
  J. Boone the short note on the use of trendlines to describe
  the system in the original paper has been extended to a more
  extensive explanation. Inserted September 3d, 2012.


Notes and references

  1. This article is a shortened version of a report in Dutch: 'Brandstofbesparing bij de Nederlandse elektriciteitsvoorziening' sent to the Netherlands Government and Parliament in August 2012. Parts usually well known to insiders have been left out.

  2. C. le Pair & K. de Groot: The impact of wind generated electricity on fossil fuel consumption.
    http://www.clepair.net/windefficiency.html

  3. K. de Groot & C. le Pair: The hidden fuel costs of wind generated electricity; Spil 263-264(2009) nr.5, 15/17 & http://www.clepair.net/windsecret.html

  4. F. Udo, K. de Groot & C. le Pair: Wind turbines as a source of electricity;
    http://www.clepair.net/windstroom%20e.html

  5. F. Udo: Wind energy in the Irish power system; http://www.clepair.net/IerlandUdo.html

  6. C. le pair: Electricity in The Netherlands; Wind turbines increase fossil fuel consumption and CO2 emission; http://www.clepair.net/windSchiphol.html

  7. F. Udo: Wind energy and CO2 emissions - 2; http://www.clepair.net/Udo-okt-e.html

  8. C. le Pair, F. Udo & K. de Groot: Windturbines as yet unsuitable as electricity providers; Europhysicsnews 43 (2012) nr.2, p. 22/5 & http://www.clepair.net/europhysics201203.html.

  9. F. Udo: Curtailment in the Irish power system; http://www.clepair.net/Udo-curtail201205.html

  10. W. Post, many contributions on The Energy Collective; http://theenergycollective.com/index.php?q=willem-post/64492/wind-energy-reduces-co2-emissions-few-percent

  11. H. Sharman: Wind Energy, the case of Danmark; http://www.CEPOS.dk; download:
    http://www.cepos.dk/fileadmin/user_upload/Arkiv/PDF/Wind_energy_-_the_case_of_Denmark.pdf

  12. P.F. Bach (on his website): http://pfbach.dk

  13. BENTEK: How less became more. Wind, Power and unintended consequences in the Colorado Energy Market, 2010. http://www.bentekenergy.com

  14. CBS, Statline: http://statline.cbs.nl/StatWeb/dome/default.aspx

  15. 1 GWy = 1 000 000 x 24 x 365 kWh. 1 kWh = 1000 x 3600 J. J = Joule. 1 TJ = 1012 J

  16. G. Dijkema, Z. Lukszo, A. Verkooijen, L. de Vries, M. Weijnen: De regelbaarheid van elektrische centrales; een quickscan i.o.v. het Ministerie van Economische zaken, 20 april 2009.

  17. http://www.energietech.info/groengas/theorie/gasmotoren.htm

  18. Priv. comm. Borssele.

  19. H.O. Voorma: Biobrandstoffen, lecture Zeist, 2012 04 18. Prof. (em) dr. Voorma, biochemist, former Rector Magnificus of Utrecht University

  20. (2), note 13.

  21. Lenzen, M.: Life cycle energy and greenhouse emissions of nuclear energy: a review; p. 137 ff. & Energy conversion and management 49 (2008) 2178-2199, download: http://www.isa.org.usyd.edu.au/. See also http://bravenewclimate.com/2009/10/18/tcase4/.